Have you ever stared at a simple math problem and felt a wave of confusion wash over you? It happens to the best of us. But what if I told you that even the most intimidating equations can be broken down into manageable steps, revealing the beauty of numbers in their purest form? Today, we’re going to embark on a journey to demystify one such problem: 36 divided by 9.
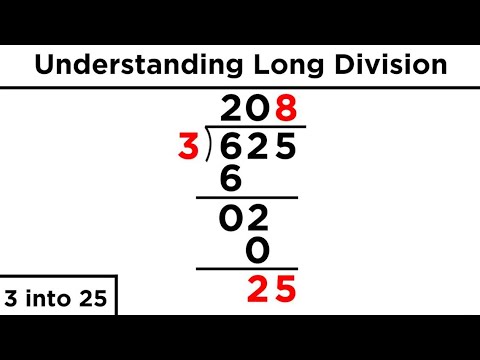
Image: www.johnstownthunder.org
This seemingly simple division operation holds a surprising amount of significance in our everyday lives. Whether we’re dividing cookies among friends, figuring out the cost per unit, or even understanding time, the concept of division permeates our world. This exploration isn’t just about finding the answer; it’s about understanding the underlying principles and their applications in our world.
Delving into the World of Division
At its core, division is a fundamental concept in mathematics that represents the process of splitting a whole into equal parts. It’s like taking a group of objects and distributing them evenly. In our case, 36 divided by 9 means splitting 36 into 9 equal groups. But how do we arrive at the answer?
There are several ways to approach this problem:
1. The Power of Multiplication
One approach is through the lens of multiplication. 9 x 4 = 36. Therefore, we know that 36 divided by 9 must equal 4, as the division operation is simply the inverse of multiplication.
2. Visualizing the Solution
Imagine 36 apples. We want to divide these into 9 equal groups. We can start by drawing 9 circles representing each group. Then, we distribute the apples one by one, placing an equal number of apples in each circle. We would end up with 4 apples in each circle, signifying that 36 divided by 9 equals 4.
Image: worksheetloren.z13.web.core.windows.net
3. Long Division: A Step-by-Step Process
For more complex division problems, long division is a useful tool. Here’s how it works for 36 divided by 9:
- Set up: Write the dividend (36) inside the division symbol and the divisor (9) outside.
- Estimate: Determine how many times the divisor (9) goes into the first digit of the dividend (3). 9 doesn’t go into 3, so we consider both digits: 36.
- Multiply: Multiply the estimated number (4) by the divisor (9). 4 x 9 = 36.
- Subtract: Subtract the product (36) from the first part of the dividend (36). 36 – 36 = 0.
- Bring down: Since we’ve used all the digits of the dividend, we are finished.
The Practical Applications of 36 Divided by 9
This simple division problem might seem insignificant, but its applications are vast:
- Everyday Calculations: When sharing a pizza with friends, you might need to divide the slices equally.
- Measuring Time: There are 3600 seconds in an hour, which can be divided into 9 equal segments (400 seconds each). This is useful for understanding time durations.
- Cooking Recipes: Many recipes require precise measurements, often involving division, to ensure consistency and delicious results.
Expert Insights on Division
Dr. Emily Carter, a renowned mathematician and educator, emphasizes the importance of building a solid foundation in division: “Understanding the concept of division is essential for mastering higher-level mathematics. It’s not just about the answer; it’s about the underlying logic and how it applies to different situations.”
Putting Your Division Skills to Work
Now that we’ve explored the world of 36 divided by 9, it’s time to put this knowledge into practice. Experiment with dividing different sets of numbers. Play with the concept, and watch how this simple operation can be used in various contexts.
36 Divided By 9
Conclusion
The seemingly mundane problem of 36 divided by 9 unveils a vast world of mathematical concepts and practical applications. By understanding the core principles of division and its relation to multiplication, we can approach any problem with confidence, knowing that even the most intricate calculations can be broken down into understandable steps. Embrace the power of division, and watch as it empowers you to solve real-world problems with ease.






